Experiment -5
Objective: To determine the angle of minimum deviation for a given prism by plotting a graph between angle of incidence and angle of deviation.
Apparatus Required:
A drawing board, a sheet of paper, a pencil, a glass triangular prism, pins, a half-meter scale, graph paper and a protractor.
Formula Used:
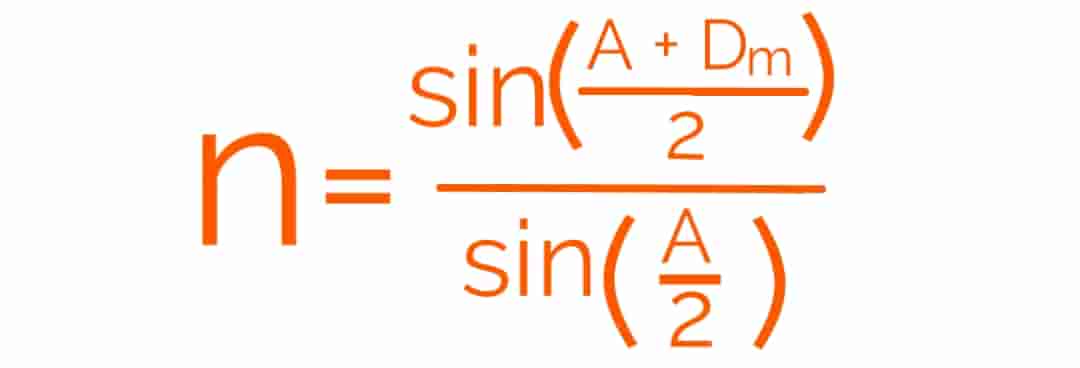
where,
n is the refractive index of the glass prism.
A is the angle of the prism
Dm is the minimum deviation.
Ray Diagram:
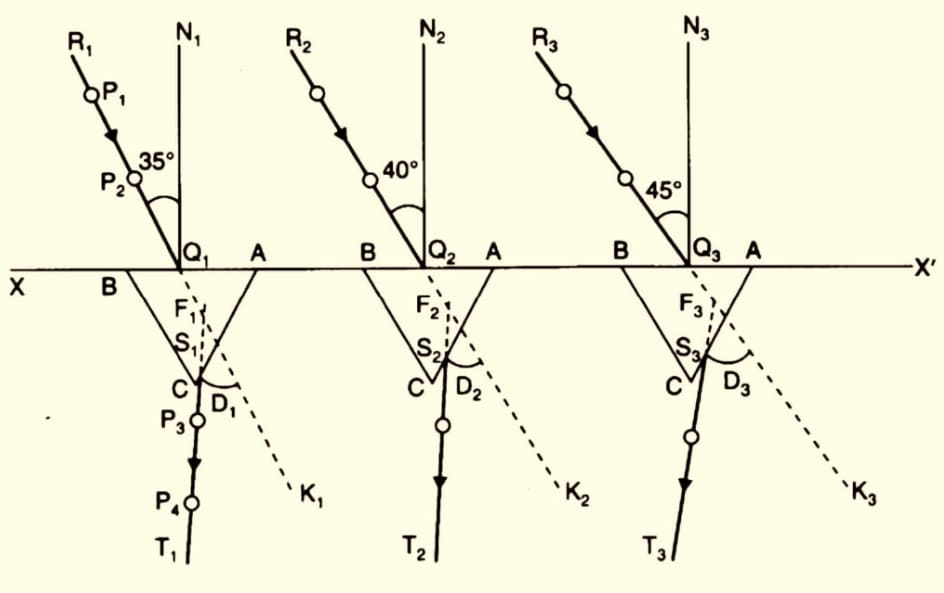
Observations:
The angle of the prism, A = 60º
Table for the angle of incidence, i and angle of deviation δ for a prism
| Serial No. | Angle of incidence (∠i) | The angle of incidence (∠i) |
|---|---|---|
| 1. | 35° | 43° |
| 2. | 40° | 39° |
| 3. | 45° | 38° |
| 4. | 50° | 38° |
| 5. | 55° | 39° |
| 6. | 55° | 42° |
Calculation:
To plot the graph between the angle of incidence ∠i and the angle of deviation ∠D, take ∠i along the x-axis and ∠D along the y-axis. Minimum deviation Dm can be found from the graph which would be corresponding to the lowest point in the graph.
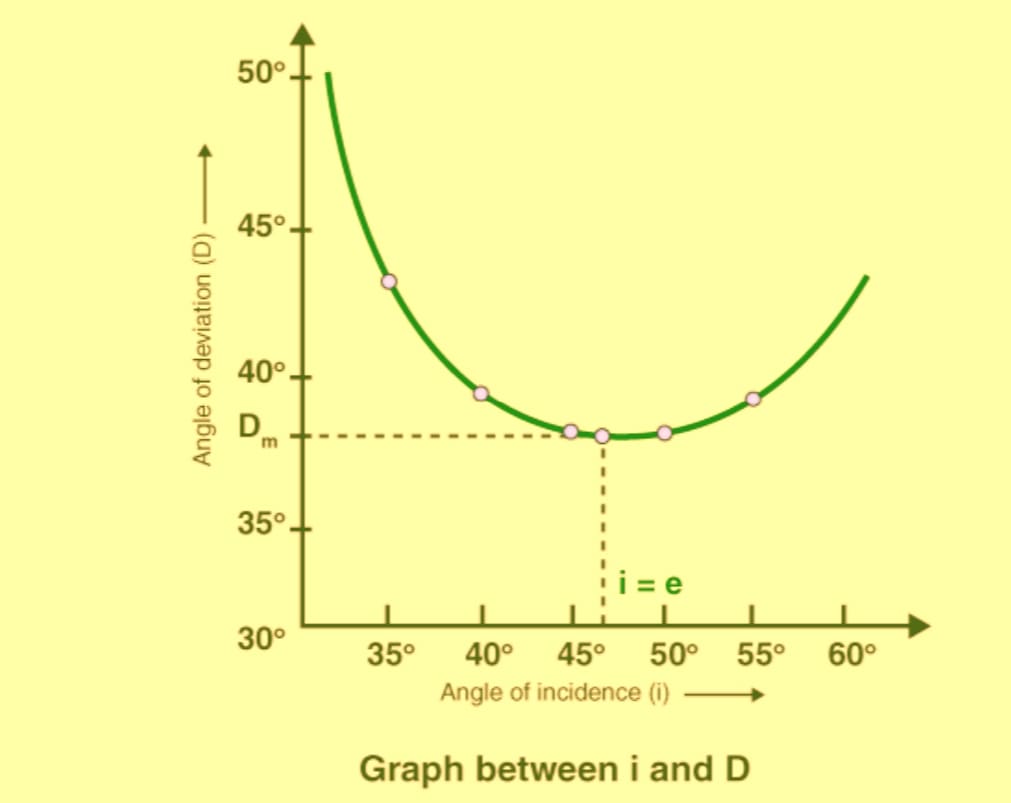
Let the value of minimum deviation, Dm= 37°
then,
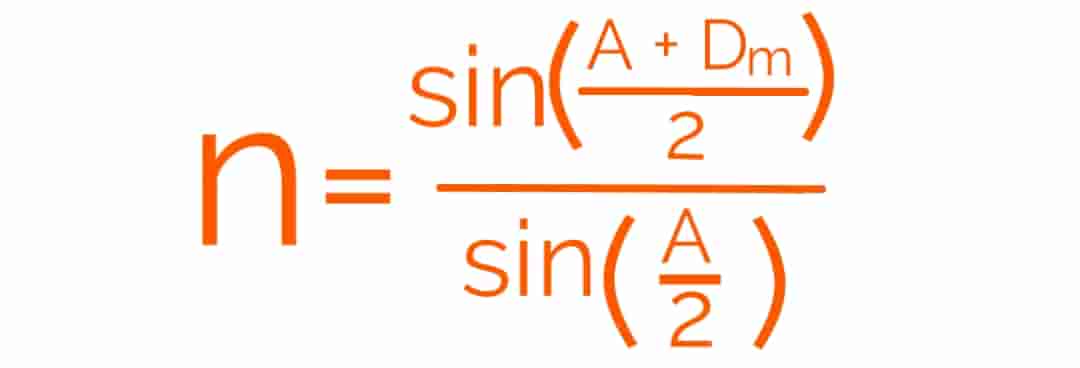
Putting the A=60° and Dm =37° in the above equation, we get n= 1.5
Result:
- Angle of minimum deviation, Dm= 37º
- the refractive index of the material of the prism, n= 1.5
- The graph indicates as the angle of incidence increases, the angle of deviation first decreases to attain the minimum value of Dm and then again increases with the angle of incidence.
Precautions:
- 35°- 60° is the angle of incidence that needs to be maintained.
- The placement of the pins must be vertical.
- The placement of two pins should be such that the distance is not more than 10mm.
- To represent incident and emergent rays, arrowheads must be marked.
- The angle of the prism used should be the same for all the observations.
Sources of error:
- The pricks made by the pin might be thick.
- Angles might be wrong while measuring them.
Viva – Voice
Q.1. Give the relation between angle of incidence i, angle of emergence e, angle of deviation D and the angle of prism A.
Ans. A+D = i+e
Q.2. What is the radius of curvature of the curved surface of the concave lens?
Ans. Clearly, it is equal to the radius of curvature of the lower surface of the convex lens.
Q.3. What happens to the frequency of light as it goes from water to glass?
Ans. It remains the same although wavelength decreases.
Q.4. What type of lens is formed between the lens and the mirror?
Ans. Plano-concave lens.
We hope the provided CBSE Class 12 Physics Practical Section-B Experiment-7 will be helpful to you. If you have any queries regarding the Class 12 Physics Practical, please drop a comment below, and we will get back to you as soon as possible. Thanks for reading this article.

